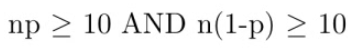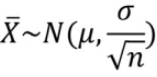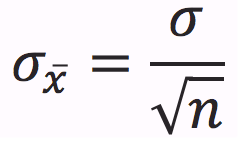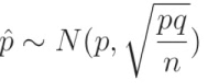Parameter vs. Statistic

, mean)

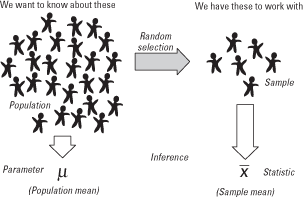
Sampling Distribution

  • The "sampling distribution" is the values taken by the statistic in all possible samples of the same size from that population

  • The "sampling distribution" is always referring to the distribution of the sample

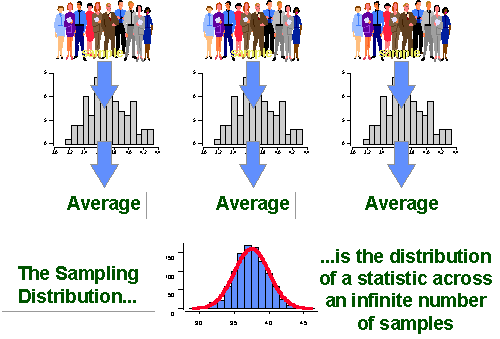
    Average me Sampling Distribution... Average Average ...is the distribution of a statistic across an infinite number of samples

Central Limit Theorem

  • The sampling distribution of the sample mean is normally distributed

    Image result for Sampling Distribution

KVl\!qeqojd%OOL 3E8 一 no P330 0 10u RI\!l\!qeqoJd Kunqeqo-ld 2m2 \>

Conditions (RIN)

  • Random

    • How the sample is selected

  • Independent

    • N≥10n

    • N: population size

    • n: sample size

  • Normal

    • For means

      • C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image168.png

      • If the population is normally distributed, n can < 30

    • For proportions:

      • np 2 10 AND n(l-p) 2 10

Sampling Distribution of a Sample Mean

  • C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image170.png

  • C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image171.png

Sampling Distribution of a Sample Proportion

  • 'd)lV

Review

Sampling Distribution Variable Categorical (example: left-handed or not) Quantitative (example: age) Parameter P = population proportion g = population mean, o = population standard deviation Statistic D — sample proportion x = sample Center Spread Shape Normal IF np 10 and n(l -p) 10 When will the distribution of sample means be approximately normal

Practice Questions

  1. Assume graph X represents the actual distribution select which graph the sampling distribution of the sample mean look like, for a sample size of n = 50?

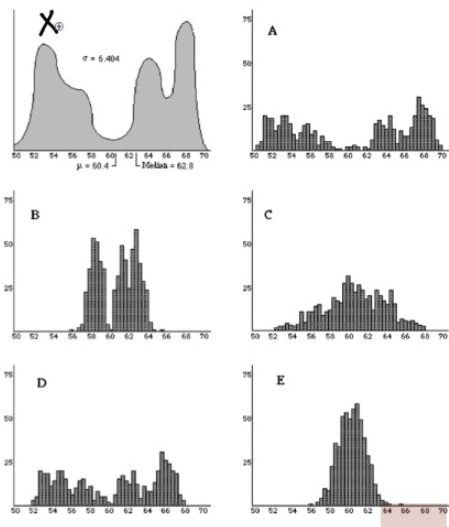
    C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image174.png

    • Answer: E
  1. The weight of the eggs produced by a certain species of chicken is Normally distributed with mean 65 g and standard deviation 5 g.

  • If a farmer selects a random sample of 10 every morning to check the health of his laying hens, what is the mean and SD of the sampling distribution of the weight of the eggs?

    70

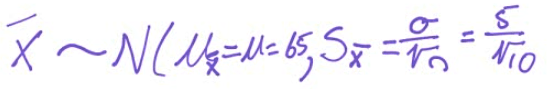
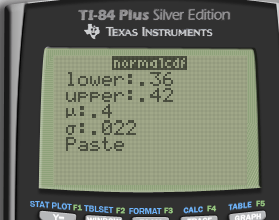
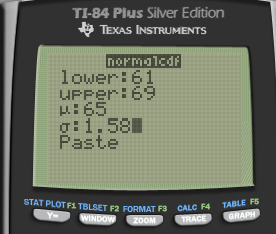
  • Calculate the probability that a randomly selected egg weighs between 61g and 69g

    Tl-84 Pius Silver Ecfitjm TEXAS INSTRUMENTS STAT FORMAT n CALC

  • Calculate the probability that the mean weight of the farmers 10 eggs falls between 61g and 69g.

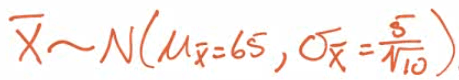
    C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image177.png

Ti-" Plus Silver Editjm TEXAS INSTRUMENTS p: 65 P ast\*

  1. A survey asks a random sample of 500 adults in California if they support an increase in the state sales tax of 1%. Suppose that 40% of all adults in California support the increase.

  • If p hat is the proportion of the sample who are in favor of the increase, what is the mean of the sampling distribution of p hat? The SD?

    C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image179.png

    -z ZO •

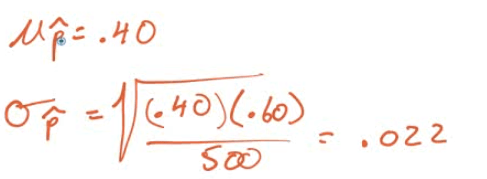
  • How large a sample would be needed to guarantee that the standard deviation of it is no more than 0.02?

    C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image181.png

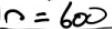
    C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image182.png

  • Find the probability that p hat is between 0.36 and 0.42

    Tl-B4 mus Silver Ecfitjm TEXAS INSTRUMENTS STAT FORMAT n CALC TABLE

results matching ""

    No results matching ""